SETI and Aesthetics
Are there any universal principles in science and aesthetics that could help us to set the unknown parameters for interstellar communication?
Guillermo A. Lemarchand
Instituto Argentino de Radioastronomia (CONICET) &
Centro de Estudios Avanzados (Universidad de Buenos Aires)
C.C. 8 - Sucursal 25; (1425) Buenos Aires, Argentina
Jon Lomberg
The Planetary Society
P.O.Box 207,
Honaunau, Hawaii 96726
ABSTRACT
The success of radio search for extraterrestrial intelligence (SETI) depends not only on the unknown abundance of civilizations in our galaxy, but also on their assumed transmission strategies. In this paper we suggest that, in order to maximize the probability of the discovery of an interstellar message, the parameters be set choosing those which minimize the number of unknown dimensions to be searched by the recipient, using those principles in science and aesthetics which may be "universally" known among intelligent civilizations. These include various symmetries, particularly radial and bilateral, and constants such as phi, the so-called "Golden Section"
I- INTRODUCTION:
Assuming that the best way for establishing contact across interstellar distances is the use of electromagnetic waves (Shklovskii and Sagan, 1966), the space of configuration for the transmission of interstellar signals includes a four dimensional sub-space [R, a, d, t] (where R† is the distance to the target star, a the right ascension, d the declination, t †the time after the transmission of the signal) and a seven dimensional information subspace [m, f, ti, ∆B, P, C, S† ] (where m †is the modulation type, f†the frequency, ti the information rate, ∆B †the spectral width of the transmitting signal, P †the polarization, C, †the code and S†the semantics.) †(Dixon 1973; Makovetskii 1980; Lemarchand 1994).
Even if there are large numbers of mutually communicative civilizations, the probability that the Earth would be able to detect an ETI beacon signal following a random addressing times strategy for transmission and reception is vanishingly small (von Hoerner, 1961; Bates, 1978). In order to be detected by an emergent civilization, the best transmission strategy should set all the variables of the space of configuration following the Principle of Anti-Cryptography (Dixon, 1973). In this way the signal should be designed and operated to maximize its probability of discovery, both by intentional searches and by accidental observations.
Scientific principles were called to propose "universal" ways in order to set the different variables for anti-cryptographic interstellar communications: best frequencies (the microwave window by Drake, 1959), best interstellar bandwidth (Cordes & Lazio, 1991); to select the targets (sky survey vs. nearby stars), the synchronization between transmissions and receptions of electromagnetic signals (Pace and Walker 1975; Tang 1976; Makovetskii 1977; McLaughlin 1977; Lemarchand 1994); the correlation between direction in the space and frequencies that could be amplify by interstellar masers (Marimoto et al. 1978; Cordes, 1993); etc.
In order to select the best strategy for transmission as well as for reception, we must look carefully at the way we can optimize all the free parameters, assuming that the anti-cryptography principle and communication by means of electromagnetic waves are the typical assumptions among galactic civilizations.
There is an assumption of symmetry underlying the hope that transmitters and recipients will arrive at similar, if not identical strategies. We hope that the strategies of senders and receivers will be symmetrical (we look where they send). We hope that our technologies will be symmetrical (we listen with machines that can detect their signals). And we hope that ideas of what constitutes "anti-cryptography" will also be symmetrical, or else we will never decode or perhaps never even recognize, an interstellar message.
We ask in this paper whether other properties of symmetries may therefore offer some advantages when setting the free parameters of our search space, since these same symmetries might be known to all intelligent species and provide mutually obvious clues as to how and where interstellar signals will be found.
II-SYMMETRY IN NATURE AND AESTHETICS
One reasons to believe that an appreciation of symmetries may be universal is to observe how widely they reappear in nature, science and in independently created human art forms. They form the basis for many patterns that occur in human art, and thus are a common principle of aesthetics. Symmetry and other aesthetic principles are deeply related to mathematics and physics. If we assume that scientific extraterrestrials share enough of our mathematics and physics on which to base communication, it is worth considering whether they might know and share some of these aesthetic principles as well. We shall discuss examples of symmetries (radial, bilateral, ellipsoidal, etc.) and one example of another aesthetic principle (phi, the Golden Section) to illustrate some concepts which may have an application in SETI.
In nature many inanimate objects and organisms exhibit obvious and remarkable bilateral, radial, concentric, and other mathematically based symmetries. In science these symmetries are important in the study of crystals, celestial mechanics, virus structure, fracture patterns in materials, mathematical topology and group theory (Weyl, 1952). And symmetries have been of† fundamental and species-wide importance in the history of human culture, in art forms including architecture, music, dance, poetry, drama, and film; and in activities like sports and games (Ghyka, 1952; †Huntley, 1970). The chessboard and the soccer field are as formally symmetrical as the mandala.
Here we present some examples of symmetries we suspect may recognized throughout the universe:
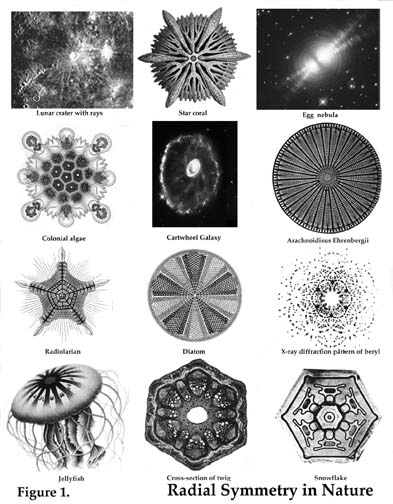
(1) Radial symmetry: Let us consider the example of radial symmetry, an arrangement based on radii emanating from a common center. In its most regular forms the radii are separated by a constant number of degrees. Radii may be of different lengths, but opposite radii are usually the same length. Looser radial symmetries with no regularity of the length and angle of radii can also be found in random processes like cratering. In Earth's biosphere radial symmetry is found throughout many phyla and kingdoms, from the shells of radiolarians to the growth patterns of colonial algae, from the †lotus flower to the †octopus, from the spider's web to the human eye.
In human art radial symmetry is one of the commonest design motifs in the world, equally obvious in the stonework of Gothic and Aztec masons, in the symbols of Chinese and Navajo people, in the religious iconography of Tibet and family crest design in Japan. Indeed, mandala patterns are among the few designs found in almost every human culture known. (see accompanying illustrations, from ArgŁelles, 1972; and Haeckel 1904)
Isotropic radiation is itself a universally occurring, three dimensional photon mandala. Many human mandalas possibly originated in a two dimensional projection of the Sun's radiation. The Big Bang can even be envisioned as a four dimensional cosmic mandala. It is interesting to note that one repeated characteristic of mandalas in human art is the necessity of having a slight flaw in the symmetry, so, it is said, the soul will not be trapped. Without some flaws, so-called anisotropies, in the symmetry of the Big Bang, galaxies and stars would never have appeared.
IMPLICATIONS FOR SETI: The implication for SETI strategies is that a commonly recognized, mutually guessable unique point will be any center common to both parties. For other civilizations in the Milky Way, that unique point is the center of the galaxy. Consider Earth to be at one location in the "galactic mandala". If there are many transmitting civilizations in the galaxy, some other civilizations may exist along the same radius as the Sun. These civilizations would therefore occupy a privileged position over other galactic locations from the point of view of symmetry. Radial symmetry thus offers a solution to the question of where in the sky interstellar beacons might preferentially be directed and found.
According to radial symmetry, beacons and the searches for them should concentrate along the radius connecting between us, the galactic center, and the galactic anti-center. There are at least 108 stars visible along this axis, so a very large number of candidate solar systems would be encompassed. By limiting the search to this axis, we increase the time we can spend on the candidate source from a few seconds to many months (a factor of 106 improvement), creating a "deep field" radio survey of that area of sky.†This may substantially increase our chances of detecting a strong, periodic beacon. Another benefit of this strategy is to simplify the problem of how to compensate for difference in relative proper motion of sender and receiver since we can use the Galactic Barycenter as a common rest of frame.
2) Omnipresence of phi : The Greeks had already noticed that three terms at least are necessary in order to express a proportion; such is the case of the continuous proportion (a/b) = (b/c). But the problem could be simplified by reducing to two the number of terms, and making c = a+b, so the continuous proportion becomes (a/b) = (b/(a+b)). By resolving the last equation we found that b = (1+ √5)/2 a or b = (1- √5)/2 a. The constant of proportionality is named phi (approximating to 1/1.61803...~ 0.61803...).
Phi has been studied since the time of Pythagoras, and variously known as the Golden Section and the Divine Proportion. In 1509 Luca Pacioli published De Devina Proportione, illustrated by Leonardo da Vinci with one of the most famous drawings in the history of Western art: a four-armed, four-legged man exhibiting the proportions of phi in his own body. Kepler (1571-1630) said of the Golden Section: "Geometry has two great treasures: one is the Theorem of Pythagoras: the other the division of a line into extreme and mean ratio. The first we may compare to a measure of gold; the second we may name a precious jewel."
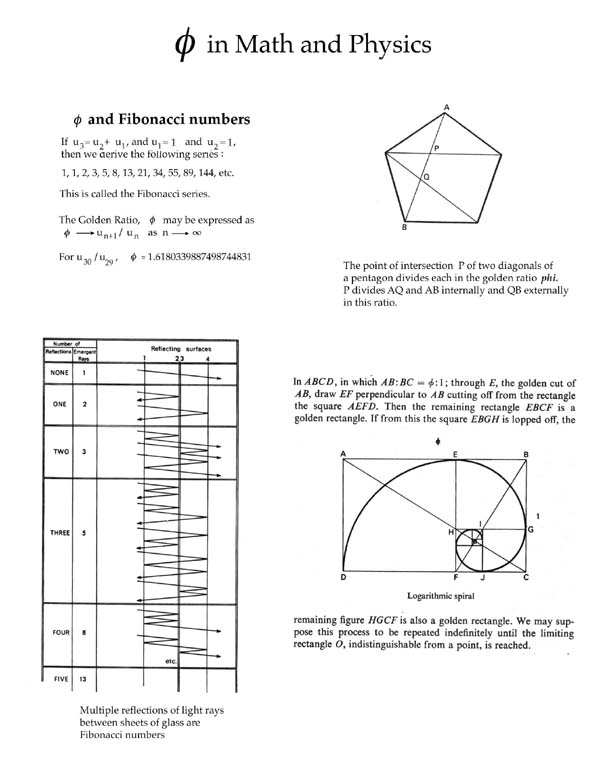
Phi may be found in mathematics by creating ratios of successive terms of the Fibonacci series (0,1,1,2,3,5,8,13,21,34....). It is also similarly derived from any other example of the Lucas series (un-1 + un = un+ 1), of which the Fibonacci is the simplest case (where n=1). It may be noted that the presence of phi in the Fibonacci series also works in all arithmetics besides base 10. Phi has very curious mathematical properties. For example, †number theory shows that phi is the most irrational number and also has the following identity
phi n = phi (n-1) + phi (n-2). These properties can be used for computational numerical algorithms for optimal methods of function minimization (Press et al., 1986).
Our treatment of phi will be very cursory, but a deeper study will reveal a large number of other cases where phi appears, besides those few enumerated here.
Phi makes many appearances in geometry: lines connecting the vertices of a pentagram form a five-pointed star, each line of which bisects two other lines, cutting each into two line segments whose proportions exhibit phi. Phi is also found in 3:4:5 right triangles, in the solution to Neville's 5-disk problem and in many other areas of mathematics.
Phi, and the Fibonacci series exhibiting phi appear in the real world in many physical and biological phenomena (Cook 1914; Thompson 1942). Here are †a few examples from physics and biology:
If a beam of light is incident upon two sheets of glass in contact, there will be multiple reflections. The number of different paths followed within the glass before the ray emerges depends on the number of reflections which the ray undergoes. The number of emergent rays is a Fibonacci number (see diagram).
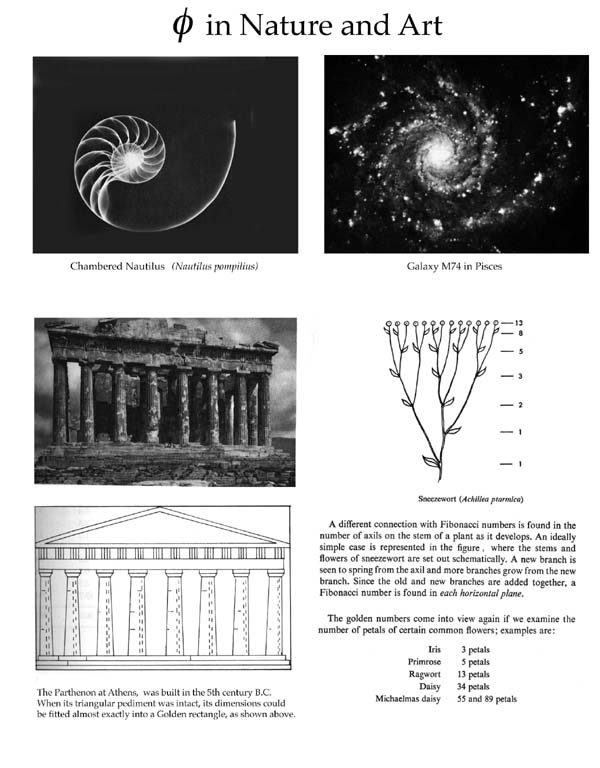
A spiral can be formed along the ends of adjacent perpendiculars whose proportion is phi. This lovely spiral is seen in some of the handsomest spiral galaxies, such as M51, M101, and M74. This spiral has been noticed by many mathematicians and been variously called the equiangular spiral and the logarithmic spiral.
Phi may be seen in biology in population growth of successive generations of rabbits; in the genealogy of the drone bee; and in the average value of leaf divergence measured on these stems of plants (this is angular separation of successive leaves on a stem as measured on a helix drawn from the root of the plant upwards in many species of plants).
The number of petals on many common flowers is a Fibonacci number (Iris-3; Primrose-5; Ragwort-13; Daisy-34) Plants with composite floret patterns, like the sunflower, the pinecone, and pineapple show overlapping clockwise and counter-clockwise spirals patterns. In the sunflower there are 21 petals in clockwise spiral, 34 in the counter-clockwise spiral; in the pine cone 5 clockwise, 8 counter-clockwise; in the pineapple 8 clockwise and 13 counter-clockwise. The number of florets in each spiral is a Fibonacci number, and they are successive Fibonacci numbers!
The equiangular spiral, generated by connecting the ends of perpendicular lines whose length increase by a factor of phi, appears in the curve of animal horns and in the seashell, Nautilus pompilius, the chambered nautilus.
Phi's ability to generate beautiful patterns has been exploited by human artists for 2000 years. The Greek sculptor Phidias used it, and the proportion of the height to the length of the Parthenon is a close approximation to phi. In the two millennium† since the Parthenon was designed phi has continued to be a touchstone for generations of graphic and design artists and architects . (Huntley 1970).
We cannot say why this "golden" proportion occurs in processes seemingly as unrelated as galactic rotation and growth patterns of flowers, horns and shells. But for whatever reason it seems to be deeply embedded in the mathematical description of phenomena throughout the real universe. It is reasonable† to assume that other intelligences studying the cosmos will have discovered this proportion as well.
IMPLICATIONS FOR SETI: The implications for SETI strategy may be that a unique and universally distinctive number is derived which can be used as a required constant wherever a specific number is required in any parameter of search space. It might be useful in anticipating the periodicity of beacons, or in selecting the magic frequencies on which to search, or perhaps to identify privileged locations in the sky (Lomberg, 1988, 1993). It can also be used to arrange pictorial binary messages (with the Arecibo message style) but ordered, for example, in a matrix of 33,552 bits (product of 144x233 -golden mean rectangle- where 144 is the only perfect square number in the Fibonacci series, while 233, the following number in that series, is also a prime number).
(3) Other Symmetries: A similar argument can be made for bilateral symmetry. It is found everywhere throughout the biosphere. Indeed the double helix of DNA is bilaterally symmetrical, as are the members of many animal phyla. It is also found in astrophysics in the bi-polar jets emerging from proto-planetary disks, accretion disks around black holes, and from the center of massive and active galaxies.
The implication for SETI strategies is that if a particular direction is selected, one should look in both directions along that axis. Some particular applications, using ellipsoids and hyperboloid symmetries to synchronize the transmissions and receptions of call signals using nearby supernova or nova explosions were discussed by Tang (1976), Makovetskii (1977, 1980) and Lemarchand (1994).
Following this bilateral ideas and the advantage of interstellar natural amplification of cosmic masers (Cordes, 1993), we can use the convergence of "magic places" (unsaturated cosmic masers sources), with "magic frequencies" (cosmic masers frequencies, especially OH at 1.67 GHz and Methanol at 6.6 GHz) with a high gain (~ e20), with the bilateral symmetry property.
There are other forms such as spirals which occur both in art and nature, and other interesting numbers which provide unique values that can set the free parameters of search space.
III-CAN AESTHETICS BE APPLIED TO SETI?
Ultimately our argument reduces to an assumption: we believe that some aesthetic principles mirror the natural world in the sense that the same mathematics can be used to describe both (Ne'eman, 1995). Indeed aesthetic principles seem to be selected on the basis of their mathematical regularity. Many formal aesthetic principles can be described mathematically (even Aristotle's "dramatic arch" in drama and literature seems to echo the trajectory of an object, in a gravitational field). Many pre-technical cultures on Earth worked using symmetry principles (Nagy 1995; Wilson 1995). Here we assume that other intelligent beings will also have aesthetic appreciation and they can use them to set different variables of our space of configuration. This can be challenged as unprovable.
However SETI has always proceeded on the identical assumption that ETI will think mathematically, and be aware of the significance of, for example, prime numbers. The advantage of using symmetries and aesthetics in SETI is that they may allow us to restrict some parameters of search space which are otherwise inconveniently large. We believe a good case can be made for the universality of these aesthetics principles, since they are so deeply related to mathematics and natural phenomena. Every argument that can be used to defend the ubiquity of mathematics and physics can be used to defend the ubiquity of these principles of aesthetics. Our conclusion is that symmetry, as well as other aesthetic considerations can contribute to the solution of general and specific problems in SETI strategy including choices among the various frequencies, directions, and times that define particular search strategies. These principles also may play an important role in the decoding of messages, should any ever be received.
IV-REFERENCES:
ArgŁelles J. and M. (1972) †Mandala,†Shambala Press, Berkeley
and London
Bates, D.R. (1978), Astrophys. Space Sci. , vol.55, p.7
Beskin, G.M. and Sannikov, †ASP Conference Series, vol.47,
p.251
Cordes, J. and Lazio, T. J. (1991); Astrophys. J., vol.376, p.123
Cordes, J. (1993), ASP Conference Series, vol.47, p.257
Cook, T.A. (1914), The Curves of Life, Constable & Co. London
Dixon, R. (1973), †Icarus, vol.20, p.187
Drake, F.D. (1959), †Sky & Telescope, vol.19, p.140
Ghyka, M. (1952), Philosophie et Mystique du Nombre, Payot, Paris
Haeckel, E. (1904), Art Forms in Nature, Dover Pub. Inc., New York
Huntley, H.E. (1970), The Divine Proportion: A Study of
Mathematical Beauty, Dover Pub. Inc. New York.
Lemarchand, G.A. (1994), †Astrophys. Space Sci., vol.214, p.209
Lomberg, J. (1988), "Preferred Directions and Intervals of
Extra-Terrestrial Beacon Signals", paper presented at †The
Planetary Society SETI Meeting, Toronto, October 1988.
Lomberg, J. (1993), ††"The Aesthetics of SETI" ASP Conference Series, vol.47, p.427
Makovetskii, P.V. (1977), †Soviet Astronomy, vol. 21(2), p.251
Makovetskii, P.V. (1980), Icarus, vol.41, p.178
McLaughlin, W.I. (1977), Icarus, vol.32, p.464
Morimoto, M. Hiribayashi, and Jugaku, J. (1978), †Nature, vol. 276,
p.694
Nagy, D. (1995), "The 2,500-year old term symmetry in science and
art, and its `missing link' between the antiquity and the modern
age", paper presented at †Symmetry: Natural and Artificial† Third
International Symmetry Congress and Exhibition of ISIS}, Washington DC, August 14-20, 1995
Ne'eman, Y. (1995); "Symmetry as a fundamental element of physical
reality", paper presented at Symmetry: Natural and Artificial
Third International Symmetry Congress and Exhibition of ISIS},
Washington DC, August 14-20, 1995
Pace, G.W. and Walker, J.C.; Nature, vol.254, p.400
Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; and Flannery, B.P.
(1986), †Numerical Recipes, Cambridge University Press.
Shklovskii, I.S. and Sagan, C. (1966), Intelligent Life in the
Universe, Holden Day Inc, San Francisco
Tang, T.B. (1976), J. Brit. Interplanet. Soc., vol.29, p.469
Thompson, D'Arcy W. (1942), On Growth and Form, Cambridge
University Press, Cambridge.
von Hoerner, S. (1961); Science, vol.134, p.1839
Weyl, H. (1952); Symmetry, Princeton University Press, Princeton.
Wilson, A.M. (1995), †The Infinite in the Finite, Oxford University
Press, Oxford.

![]()
Return to Articles
All prices quoted in US dollars.
Copyright ©2010 Jon Lomberg. All Rights Reserved.

